Example 1. The IVP $F(x,y,y^{\text{'}})=\left|y^{\text{'}}\right|+\left|y\right|=0,y\left(0\right)=1$ has no (zero) solutions.

Example 2. The IVP $y^{\text{'}}=f(x,y)=2x,y\left(0\right)=c$ has one solution, $y\left(x\right)=x^2+c$ for any given $c$.

Example 3. The IVP $y^{\text{'}}=f(x,y)=n{y}^{(n-1)/n},n\in \{2,3,\dots \},y\left(0\right)=0$ has two solutions:

1. $y\left(x\right)=0$, and

2. $y\left(x\right)=x^n$

Example 4. The IVP $y^{\text{'}}=(y-1)/x,y\left(0\right)=1$ has infinitely many solutions, $y=1+cx$.

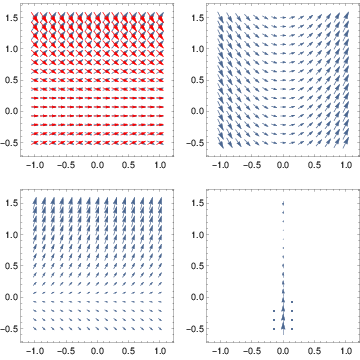Figure 1. Example direction fields