MATH529: L08 Laplace Equation

Overview

• Problem statement

• Separation of variables

• General solution

• Boundary value problems:

  • Mixed Neumann-Dirichlet

  • Dirichlet

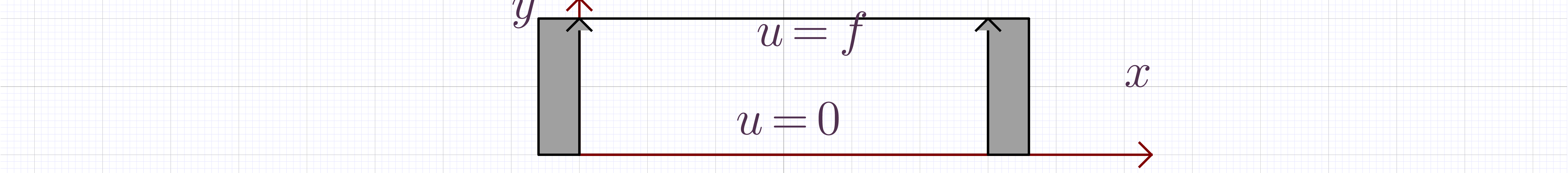
  • Inhomogeneous Dirichlet

Problem statement

• Steady-state temperature or static deformation in a plate of size $a\times b$

  ${\displaystyle \begin{array}{ll}{u}_t=k(u_{xx}+u_{yy})& u_{tt}=a^2(u_{xx}+u_{yy})\\ u_{xx}+u_{yy}=0& u_{xx}+u_{yy}=0\end{array}}$

• Laplace equation

  ${\displaystyle \frac{{\partial }^{2}u}{\partial x^2}+\frac{{\partial }^{2}u}{\partial y^2}=0}$

• Boundary conditions: thermally isolated at $x=0$, $x=a$. Fixed temperature at $y=0$, $y=b$.

  ${\displaystyle u_x(0,y)=0,u_x(a,y)=0,u(x,0)=0,u(x,b)=f\left(x\right)}$

Separation of variables

• $u_{xx}+u_{yy}=0$, $u(x,0)=0$, $u(x,b)=f\left(x\right)$, $u_x(0,y)=0$, $u_x(a,y)=0$.

• $u(x,y)=X\left(x\right)Y\left(y\right)\Rightarrow$ $X^{\text{'}\text{'}}Y+X{Y}^{\text{'}\text{'}}=0$

  ${\displaystyle \frac{X^{\text{'}\text{'}}}{X}=-\frac{Y^{\text{'}\text{'}}}{Y}=-\lambda }$

• Implications of separation of variables on boundary conditions

  ${\displaystyle \begin{array}{l}u(x,0)=X\left(x\right)Y\left(0\right)=0\Rightarrow Y\left(0\right)=0,\\ u_x(0,y)=X^{\text{'}}\left(0\right)Y\left(y\right)=0\Rightarrow X^{\text{'}}\left(0\right)=0,\\ u_x(a,y)=X^{\text{'}}\left(a\right)Y\left(y\right)=0\Rightarrow X^{\text{'}}\left(a\right)=0\end{array}}$

• Consider Sturm-Liouville problem $X^{\text{'}\text{'}}+\lambda X=0,X^{\text{'}}\left(0\right)=0,X^{\text{'}}\left(a\right)=0$

  • $\lambda =0\Rightarrow X^{\text{'}\text{'}}=0\Rightarrow X=c_1+c_{2}x$. $X^{\text{'}}\left(0\right)=c_2=0$, $X^{\text{'}}\left(a\right)=c_2=0$. $X\left(x\right)=c_1$. $u(x,y)=c_{1}Y\left(y\right)$. $Y^{\text{'}\text{'}}=0\Rightarrow Y\left(y\right)=c_3+c_{4}y$, $Y\left(0\right)=c_3=0$. $Y\left(y\right)=c_{4}y$. $u(x,b)=c_{1}Y\left(b\right)=c_1{c}_{4}b=f\left(x\right)$

Separation of variables

• $u_{xx}+u_{yy}=0$, $u(x,0)=0$, $u(x,b)=f\left(x\right)$, $u_x(0,y)=0$, $u_x(a,y)=0$.

• $u(x,y)=X\left(x\right)Y\left(y\right)\Rightarrow$ $X^{\text{'}\text{'}}Y+X{Y}^{\text{'}\text{'}}=0$

  ${\displaystyle \frac{X^{\text{'}\text{'}}}{X}=-\frac{Y^{\text{'}\text{'}}}{Y}=-\lambda }$

• Consider Sturm-Liouville problem $X^{\text{'}\text{'}}+\lambda X=0,X^{\text{'}}\left(0\right)=0,X^{\text{'}}\left(a\right)=0$

  • $\lambda ={\alpha }^2>0\Rightarrow X\left(x\right)=c_1\cos (\alpha x)+c_2\sin (\alpha x)$. $X^{\text{'}}\left(0\right)=\alpha c_2=0\Rightarrow c_2=0$. $X^{\text{'}}\left(a\right)=-\alpha c_1\sin (\alpha a)=0\Rightarrow {\alpha }_{n}a=n\pi \Rightarrow {\alpha }_n=n\pi /a$.

    $Y^{\text{'}\text{'}}-{\alpha }^{2}Y=0\Rightarrow Y\left(y\right)=c_3\cosh (\alpha y)+c_4\sinh (\alpha y).Y\left(0\right)=c_3=0$.

    $u(x,b)=X\left(x\right)Y\left(b\right)=f\left(x\right)\Rightarrow {\sum }_{n=1}^{\infty }{A}_n\cos (\frac{n\pi }{a}x)\sinh (\frac{n\pi }{a}b)=f\left(x\right)$

Separation of variables

• $u_{xx}+u_{yy}=0$, $u(x,0)=0$, $u(x,b)=f\left(x\right)$, $u_x(0,y)=0$, $u_x(a,y)=0$.

• $u(x,y)=X\left(x\right)Y\left(y\right)\Rightarrow$ $X^{\text{'}\text{'}}Y+X{Y}^{\text{'}\text{'}}=0$

  ${\displaystyle \frac{X^{\text{'}\text{'}}}{X}=-\frac{Y^{\text{'}\text{'}}}{Y}=-\lambda }$

• Implications of separation of variables on boundary conditions

  ${\displaystyle \begin{array}{l}u(x,0)=X\left(x\right)Y\left(0\right)=0\Rightarrow Y\left(0\right)=0,\\ u_x(0,y)=X^{\text{'}}\left(0\right)Y\left(y\right)=0\Rightarrow X^{\text{'}}\left(0\right)=0,\\ u_x(a,y)=X^{\text{'}}\left(a\right)Y\left(y\right)=0\Rightarrow X^{\text{'}}\left(a\right)=0\end{array}}$

• Consider Sturm-Liouville problem $X^{\text{'}\text{'}}+\lambda X=0,X^{\text{'}}\left(0\right)=0,X^{\text{'}}\left(a\right)=0$

  • $\lambda =-{\alpha }^2<0\Rightarrow X\left(x\right)=c_1\cosh (\alpha x)+c_2\sinh (\alpha x)$. $X^{\text{'}}\left(0\right)=\alpha c_2=0\Rightarrow c_2=0$, $X^{\text{'}}\left(a\right)=\alpha c_1\sinh (\alpha a)=0$. No non-trivial solution. $✠$

Solution to mixed-BC problem

• $u_{xx}+u_{yy}=0$, $u(x,0)=0$, $u(x,b)=f\left(x\right)$, $u_x(0,y)=0$, $u_x(a,y)=0$.

  ${\displaystyle u(x,y)=\sum _{n=1}^{\infty }{A}_n\cos ({\alpha }_{n}x)\sinh ({\alpha }_{n}y)}$ ${\displaystyle u(x,b)=\sum _{n=1}^{\infty }{A}_n\cos ({\alpha }_{n}x)\sinh ({\alpha }_{n}b)=f\left(x\right)}$ ${\displaystyle A_n\sinh \left({\alpha }_{n}h\right)=(f\left(x\right),\cos \left({\alpha }_{n}x\right))=\frac{2}{a}\underset{0}{\overset{a}{\int }}f\left(x\right)\cos (\frac{n\pi }{a}x)dx\Rightarrow }$ ${\displaystyle A_n=\frac{2}{a\sinh \left({\alpha }_{n}h\right)}\underset{0}{\overset{a}{\int }}f\left(x\right)\cos (\frac{n\pi }{a}x)dx.}$

Dirichlet problem

• $u_{xx}+u_{yy}=0$, $u(x,0)=0$, $u(x,b)=f\left(x\right)$, $u(0,y)=0$, $u(a,y)=0$.

• $u(x,y)=X\left(x\right)Y\left(y\right)\Rightarrow$ $X^{\text{'}\text{'}}Y+X{Y}^{\text{'}\text{'}}=0$

  ${\displaystyle \frac{X^{\text{'}\text{'}}}{X}=-\frac{Y^{\text{'}\text{'}}}{Y}=-\lambda }$

• Implications of separation of variables on boundary conditions

  ${\displaystyle \begin{array}{l}u(x,0)=X\left(x\right)Y\left(0\right)=0\Rightarrow Y\left(0\right)=0,\\ u(0,y)=X\left(0\right)Y\left(y\right)=0\Rightarrow X\left(0\right)=0,\\ u_{}(a,y)=X\left(a\right)Y\left(y\right)=0\Rightarrow X\left(a\right)=0\end{array}}$

• Consider Sturm-Liouville problem $X^{\text{'}\text{'}}+\lambda X=0,X\left(0\right)=0,X\left(a\right)=0$

  • $\lambda =0\Rightarrow X^{\text{'}\text{'}}=0\Rightarrow X\left(x\right)=c_1+c_{2}x.$ $X\left(0\right)=c_1=0$. $X\left(a\right)=c_{2}a=0\Rightarrow c_2=0$

Dirichlet problem (cont)

• $u_{xx}+u_{yy}=0$, $u(x,0)=0$, $u(x,b)=f\left(x\right)$, $u(0,y)=0$, $u(a,y)=0$.

• $u(x,y)=X\left(x\right)Y\left(y\right)\Rightarrow$ $X^{\text{'}\text{'}}Y+X{Y}^{\text{'}\text{'}}=0$

  ${\displaystyle \frac{X^{\text{'}\text{'}}}{X}=-\frac{Y^{\text{'}\text{'}}}{Y}=-\lambda }$

• Implications of separation of variables on boundary conditions

  ${\displaystyle \begin{array}{l}u(x,0)=X\left(x\right)Y\left(0\right)=0\Rightarrow Y\left(0\right)=0,\\ u(0,y)=X\left(0\right)Y\left(y\right)=0\Rightarrow X\left(0\right)=0,\\ u_{}(a,y)=X\left(a\right)Y\left(y\right)=0\Rightarrow X\left(a\right)=0\end{array}}$

• Consider Sturm-Liouville problem $X^{\text{'}\text{'}}+\lambda X=0,X\left(0\right)=0,X\left(a\right)=0$

  • $\lambda =-{\alpha }^2<0\Rightarrow X\left(x\right)=c_1\cosh (\alpha x)+c_2\sinh (\alpha x)$. $X\left(0\right)=c_1=0$. $X\left(a\right)=c_2\sinh (\alpha a)=0\Rightarrow c_2$ $✠$

Dirichlet problem (cont)

• $u_{xx}+u_{yy}=0$, $u(x,0)=0$, $u(x,b)=f\left(x\right)$, $u(0,y)=0$, $u(a,y)=0$.

• Consider Sturm-Liouville problem $X^{\text{'}\text{'}}+\lambda X=0,X\left(0\right)=0,X\left(a\right)=0$

  • $\lambda ={\alpha }^2>0\Rightarrow X\left(x\right)=c_1\cos (\alpha x)+c_2\sin (\alpha x)$. $X\left(0\right)=c_1=0$. $X\left(a\right)=c_2\sin (\alpha a)=0\Rightarrow {\alpha }_{n}a=n?\pi$.

    $Y^{\text{'}\text{'}}-{\alpha }^{2}Y=0\Rightarrow Y\left(y\right)=c_3\cosh (\alpha y)+c_4\sinh (\alpha y)$.

    $u(x,0)=X\left(x\right)Y\left(0\right)=X\left(x\right)c_3=0\Rightarrow c_3=0$

    $u(x,b)=f\left(x\right)=X\left(x\right)Y\left(b\right)=X\left(x\right)c_4\sinh (\alpha b)$

    ${\displaystyle A_n=\frac{2}{a\sinh \left({\alpha }_{n}b\right)}\underset{0}{\overset{a}{\int }}f\left(x\right)\sin ({\alpha }_{n}x)dx}$
  ${\displaystyle u(x,y)=\sum _{n=1}^{\infty }{A}_n\sin ({\alpha }_{n}x)\sinh ({\alpha }_{n}y)}$

Dirichlet problem with inhomogeneous conditions on all sides

• $u_{xx}+u_{yy}=0$, $u(x,0)=f\left(x\right)$, $u(x,b)=F\left(x\right)$, $u(0,y)=g\left(y\right)$, $u(a,y)=G\left(y\right)$.

• Superposition of solution to two problems:

  • $u_{xx}+u_{yy}=0$, $u(x,0)=0$, $u(x,b)=0$, $u(0,y)=g\left(y\right)$, $u(a,y)=G\left(y\right)$ has solution $u_1(x,y)$

  • $u_{xx}+u_{yy}=0$, $u(x,0)=f\left(x\right)$, $u(x,b)=F\left(x\right)$, $u(0,y)=0$, $u(a,y)=0$ has solution $u_2(x,y)$

• The $u(x,y)=u_1(x,y)+u_2(x,y)$

  ${\displaystyle ▵u=▵u_1+▵u_2=0}$ ${\displaystyle u(x,0)=u_1(x,0)+u_2(x,0)=u_2(x,0)=f\left(x\right)}$