MATH76110/26/2018

We consider the steady-state version of the problem in Homework02, solve the resulting Helmoholtz equation using a finite element method, and compare with the analytical solution obtained by sepration of variables.

Problem.

Wave scattering by an elastic sphere submerged in an incompressible fluid is described by the equation

${\displaystyle \rho {\partial }^2𝒖/\partial t^2=(\lambda +2\mu )\nabla (\nabla \cdot 𝒖)-\mu \nabla \times (\nabla \times 𝒖)}$

(1)

where:

• $𝒖(t,𝒙)$ is the displacement [m]

• $\rho$ is the density [kg/m${}^3$]

• $\lambda ,\mu$ are the bulk, shear elastic moduli [N/m${}^2$].

Elastic media sustain two types of waves:

• longitudinal or $P$-waves (pressure waves) with wave velocity $c_p=\sqrt{(\lambda +2\mu )/\rho }$

• transverse or $S$-waves (shear waves) with wave velocity $c_s=\sqrt{\mu /\rho }$

Consider a plane pressure wave $p\left(t\right)=P\sin \left(\omega t\right)$, $$entering a cube of side $a=8$m filled with water ($\rho =1000$ kg/m${}^3$, $c_p=1500$ m/s, $c_s=0$) containing a steel sphere of radius $r=1$m ($\rho =7700$ kg/m${}^3$, $c_p=6000$ m/s, $c_s=3200$ m/s) (Fig. 1)

Figure 1. Schematic of sphere scattering problem

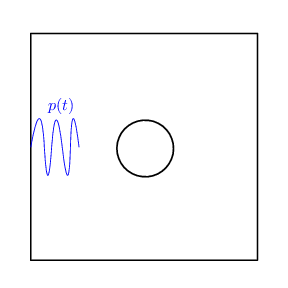Consider the Helmoholtz decomposition $𝒖=-\nabla \Psi +\nabla \times 𝑨$. The time evolution equations for $\Psi ,𝑨=(0,0,A)$ are

${\displaystyle {\Psi }_{tt}-c_p^2{\nabla }^2\Psi =0,A_{tt}-c_s^2{\nabla }^{2}A=0.}$

Looking for solutions of form $(\Psi ,A)=(\psi ,a)\exp (i\omega t)$ leads to the Helmholtz equations

${\displaystyle ({\nabla }^2+k^2)\psi =0,({\nabla }^2+l^2)a=0,k=c_p/\omega ,l=c_s/\omega .}$

The above formulations are solved in the same cases considered in Homework04

${\displaystyle }$

1. Solve the above Helmholtz problems in 2D plane geometry using FreeFEM.

2. As above, scattering around a cylinder.

3. As above, scattering around a sphere.